I love logarithms, and I'm kinda sad to be finished with them.
As I went to file away my master copies of foldables and worksheets for this unit, I was reminded of just how much I love logarithms. I don't remember doing this, but I apparently drew a smiley face on my logarithms folder when I labeled it.
 |
| Logarithms Folder |
I've already posted about the
foldables and activities I used to introduce the concept of a logarithm. Here are our last two pages regarding logarithms.
 |
| Logarithm Interactive Notebook Pages |
Properties of Logarithms will not be on my students' EOI exam at the end of the year, but I still wanted to make sure they had exposure to them.
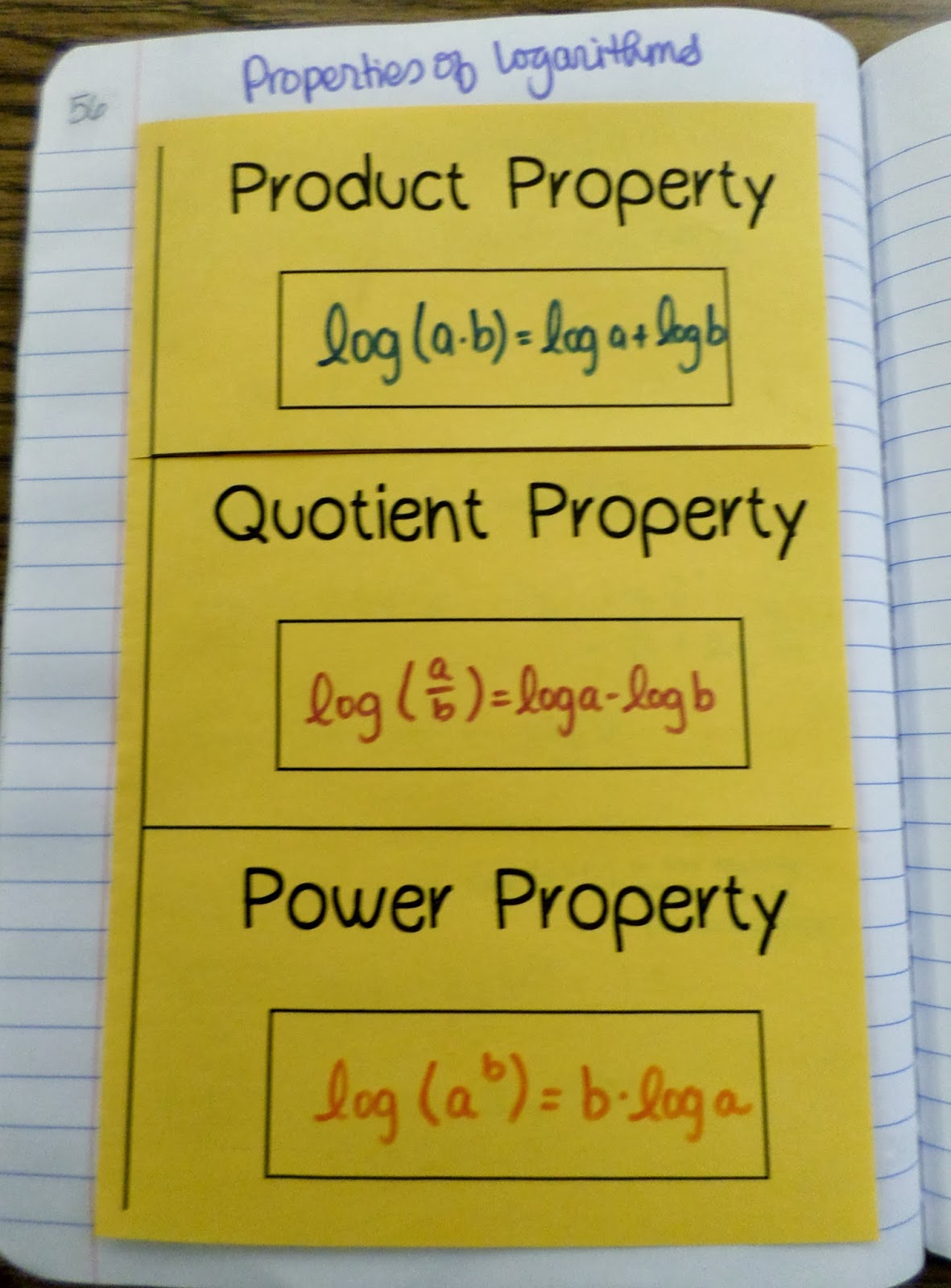 |
| Properties of Logarithms Foldable - Outside |
I projected various logarithmic expressions on the board. Students had to decide which property we could use to rewrite the expression. We opened up the appropriate flap of our foldable and recorded the example and our rewrite of the example under the flap.
 |
| Properties of Logarithms Foldable - Inside |
Our end of year exam also requires students to be familiar with the change of base formula. Last year, I used TI-84 graphing calculators with my students. We had to use the change of base formula in order to evaluate logarithms that were not written with base 10. This year, I am using TI-Nspire graphing calculators with my students. When students press the log button, they are allowed to type in whatever base they choose.
 |
| Change of Base Formula Notes - INB |
As a class, we brainstormed ways to remember the change of base formula. One student suggested that we think of the a as the attic and the b as the basement. The attic is the top level of a house. The basement is the bottom level of a house. If we are rewriting the log base b of a, the logarithm of the attic ends up on the top of our fraction. The logarithm of the basement ends up on the bottom of our fraction.
Another student suggested that was too complicated. The base of the logarithm is already written lower than the rest of the problem. So, it makes sense for the logarithm of the base to end up on the bottom of the fraction when using the change of base formula.
 |
| Change of Base Formula - INB Notes |
One other thing that we discussed was natural logarithms with a base of e. We did a short exploration of the number e on our calculators. Next year, I definitely want to spend an extra day on natural logarithms. And, I think we need to create a page regarding natural logarithms for our interactive notebooks.
Logarithm Property Foldable Template can be downloaded below:





